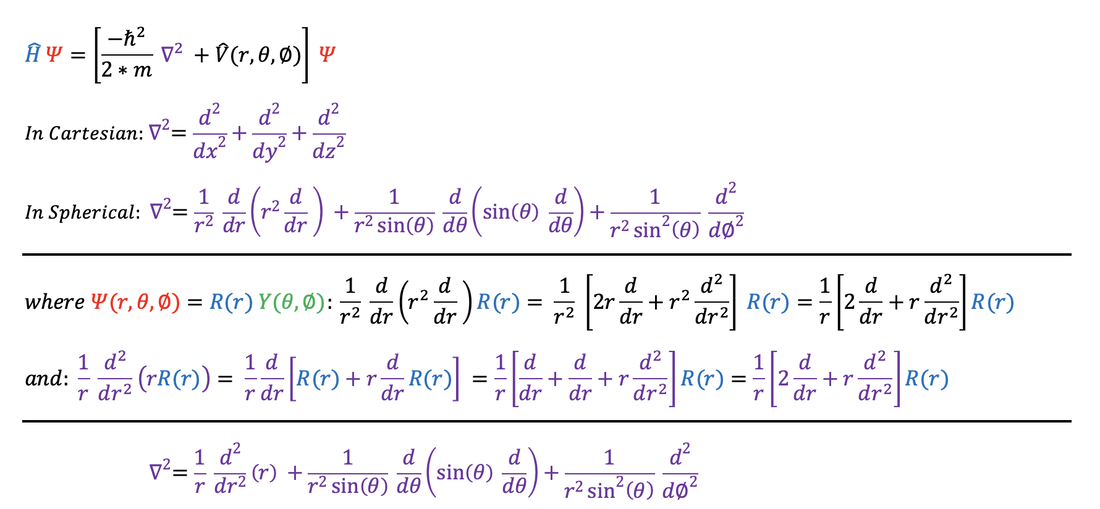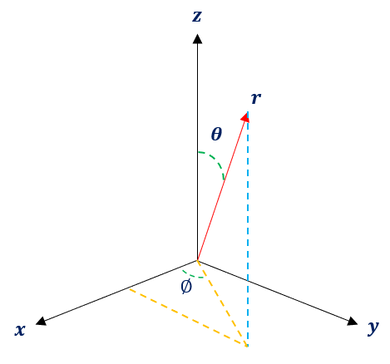
Now that we have started solving quantum mechanical problems in Cartesian coordinates, it is important to remember that such a coordinate system was completely arbitrarily chosen. There are a few ways mathematicians can describe 3D space, and Cartesian coordinates encompass only one of those ways. Another way, and a common one used for atomic models, is Spherical coordinates. We define our Spherical coordinates as follows:

          It is important to note that even among scientists, people tend to define these coordinates differently. But as long as we are internally consistent, it is okay. To be clear, my coordinates are defined as follows:
       Theta: The angle the point makes with the z axis
       Phi: The angle the point makes with the x-axis in the x-y plane
       r: the distance between the point and the origin (Some call it 'Rho')

We are now ready to redefine our Schrödinger equation below:

          The purple upside down triangle is what we call the Laplacian. It has numerous mathematical applications (the gradient for example). We previously discussed its Cartesian form. With a page worth of math, one can reduce it to its spherical form. The nice thing about the Schrödinger equation is that the Laplacian was the only explicit Cartesian form we had to change. The only other change we need to make to the Schrödinger equation is that V(x, y, z) is now V(r, theta, phi).