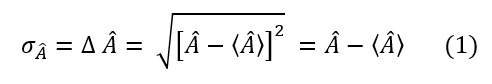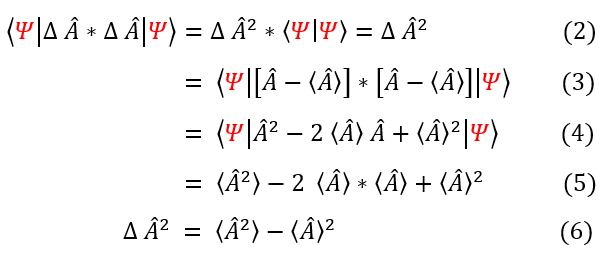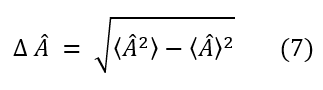An annoying part about quantum mechanics is the lack of meaning of the wave function and some postulates we take for granted. In order to gain useful information despite all of these assumptions, it is important to understand the uncertainty we hold in any result. Mathematically, we represent this uncertainty with the standard deviation.
The standard deviation of a system is found in 2 steps. First, the deviation from the average is squared (this gives us the variance). Next, we take the square root of the variance (this gives us the standard deviation). Mathematically, we represent the deviation as so:
The standard deviation of a system is found in 2 steps. First, the deviation from the average is squared (this gives us the variance). Next, we take the square root of the variance (this gives us the standard deviation). Mathematically, we represent the deviation as so:
Notation note: for some operator A, △ A represent the standard deviation of the operator (though from previous experience, some may be familiar with the sigma term). <A> represents the average value (expectation value) of the operator in its defined basis.
Because uncertainty is a physical value, we will always find the uncertainty to be a real number. Additionally, since we have a square root in the equation, the uncertainty will always be represented as a positive number.
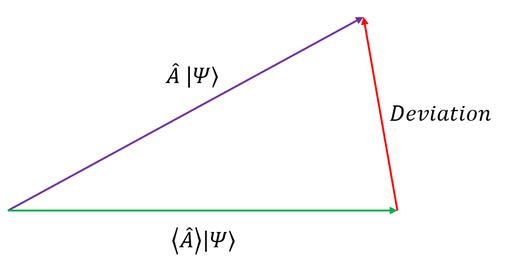
We can pictorially represent the uncertainty as so:
Because uncertainty is a physical value, we will always find the uncertainty to be a real number. Additionally, since we have a square root in the equation, the uncertainty will always be represented as a positive number.
We can pictorially represent the uncertainty as so:
While equation 1 represents the intuitive form of uncertainty, there is a simpler way to write it using only expectation values (which are super nice). This common form for the variance (standard deviation squared) is derived below:
In order to make sure everyone is following, let us review some key steps below:
2: The uncertainty is just a scalar. Applying the bra and ket to both sides (for a normalized psi) won't change the value
3: Applying the bra and ket to the operator form of the variance
3 to 4: Expanding the equation. Note: operators do not always commute, but all scalars (average values) commute
4 to 5: Apply the bra and ket to every term, noting that the expectation values are scalars (brought out of integral)
5 to 6: Simplify
Hence, the common form of the standard deviation (which we will for now on call uncertainty) is:
2: The uncertainty is just a scalar. Applying the bra and ket to both sides (for a normalized psi) won't change the value
3: Applying the bra and ket to the operator form of the variance
3 to 4: Expanding the equation. Note: operators do not always commute, but all scalars (average values) commute
4 to 5: Apply the bra and ket to every term, noting that the expectation values are scalars (brought out of integral)
5 to 6: Simplify
Hence, the common form of the standard deviation (which we will for now on call uncertainty) is:
|
|
|