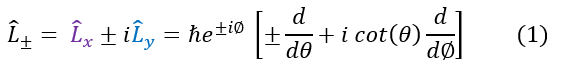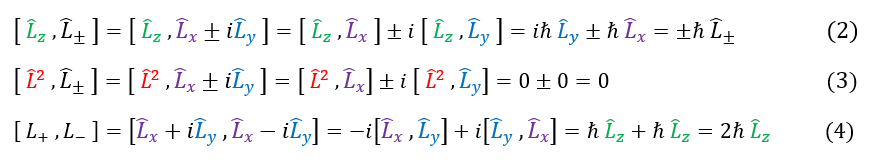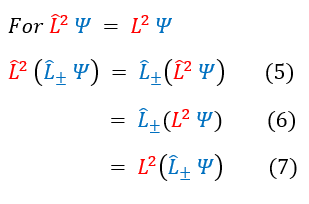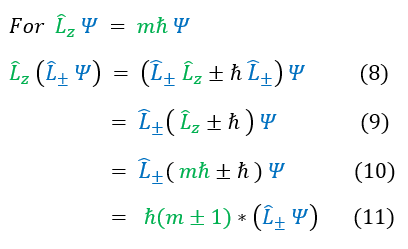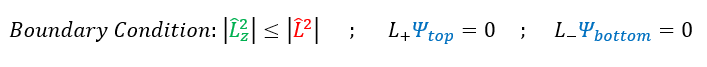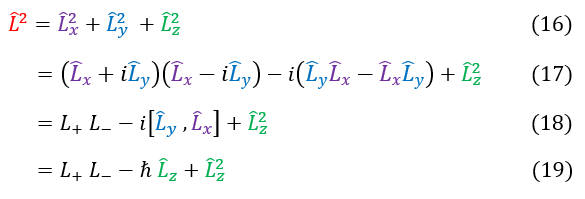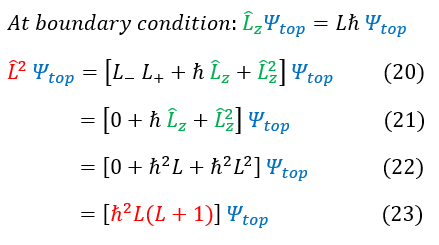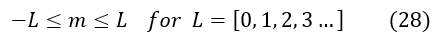Mind Network - Samuel Solomon
Angular Momentum
Ladder Operators
|
|
|
We will now discuss the angular momentum ladder operators. Just like the quantum harmonic oscillator ladder operators, and any other operator for that matter, the operator has no physical significance besides for its ability to scale an eigenfunction by a significant value. Since there is no physical motivation, we will first just plainly define the operators and later show its quantum significance. The angular momentum ladder operators are as follows:
Where 'L+' is called the raising operator and 'L-' is called the lowering operator. We previously found the spherical representations of the L_x and L_y operators. Plugging them in will lead to the spherical representation of the ladder operators on the right.
Now that we have defined this mathematical function, let us start to analyze it. First, let us see how it commutes with the regular angular momentum operators.
Now that we have defined this mathematical function, let us start to analyze it. First, let us see how it commutes with the regular angular momentum operators.
With this mathematical framework for how ladder operators work with other angular momentum operators, let us start to understand how they effect the wave function:
In order to make sure everyone is following, let us review some key steps below:
We start with the general eigenvalue definition of the 'angular momentum squared' operator on some eigenfunction.
5: We apply the same operator to an eigenfunction that has been effected by the ladder operator. Since the L^2
operator commutes with the ladder operators (see equation 3) we can switch the order of the operators.
5 to 6: We replace the L^2 operator with its corresponding (defined) eigenvalue
6 to 7: An eigenvalue is a scalar, which commutes with all operators and hence can be brought out
We perform the exact same steps on the right side with the z-component angular momentum operator. Except, now the two operators don't commute. To get around this, in equation 6 with use the commutator relationship in equation 2 to switch the order of the two operators.
Equation 6 analysis:
We notice that the ladder operator on the wave function is still an eigenfunction of the angular momentum squared operator. In fact, it even has the exact same eigenvalue 'L^2.' Eigenvalues produced from operators represent physical observables of the system. Before the ladder operator 'touched' our system, the angular momentum of the system was found to be 'L.' After the ladder operator acted on our system, the angular momentum still is 'L.' Physically, this means that the ladder operators DO NOT change the angular momentum of the system.
Equation 10 analysis:
We notice that the ladder operator on the wave function is still an eigenfunction of the z-component angular momentum operator. When previously the wave function's z-component angular momentum was 'm h_bar,' after the ladder operator acted on the wave function, this value changed. The raising operator increases the L_z of the system by h_bar and the lowering operator decreases the L_z of the system by h_bar.
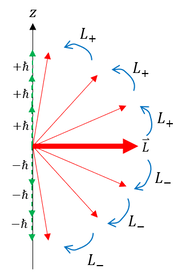
We can now physically say what the ladder operators do. Without changing the total angular momentum, it increases or decreases the z-projection / component of the total angular momentum. This can only physically happen if the total angular momentum vector rotates to either align with more or against the z-axis. We can pictorially depict this below on the left:
We start with the general eigenvalue definition of the 'angular momentum squared' operator on some eigenfunction.
5: We apply the same operator to an eigenfunction that has been effected by the ladder operator. Since the L^2
operator commutes with the ladder operators (see equation 3) we can switch the order of the operators.
5 to 6: We replace the L^2 operator with its corresponding (defined) eigenvalue
6 to 7: An eigenvalue is a scalar, which commutes with all operators and hence can be brought out
We perform the exact same steps on the right side with the z-component angular momentum operator. Except, now the two operators don't commute. To get around this, in equation 6 with use the commutator relationship in equation 2 to switch the order of the two operators.
Equation 6 analysis:
We notice that the ladder operator on the wave function is still an eigenfunction of the angular momentum squared operator. In fact, it even has the exact same eigenvalue 'L^2.' Eigenvalues produced from operators represent physical observables of the system. Before the ladder operator 'touched' our system, the angular momentum of the system was found to be 'L.' After the ladder operator acted on our system, the angular momentum still is 'L.' Physically, this means that the ladder operators DO NOT change the angular momentum of the system.
Equation 10 analysis:
We notice that the ladder operator on the wave function is still an eigenfunction of the z-component angular momentum operator. When previously the wave function's z-component angular momentum was 'm h_bar,' after the ladder operator acted on the wave function, this value changed. The raising operator increases the L_z of the system by h_bar and the lowering operator decreases the L_z of the system by h_bar.
We can now physically say what the ladder operators do. Without changing the total angular momentum, it increases or decreases the z-projection / component of the total angular momentum. This can only physically happen if the total angular momentum vector rotates to either align with more or against the z-axis. We can pictorially depict this below on the left:
|
As one can see on the left: as we act on the wave function with the ladder operator, we either raise or lower the z-component of the angular momentum by 'h_bar.' Mathematically, we are increasing or decreasing the scalar 'm' by 1. We can therefore take this scalar 'm' as a reference to the z-component of the angular momentum (and the total angular momentum by extension). Most people reference this 'm' value as 'm_L.' A slightly related quantum number is the intrinsic angular momentum's 'm' eigenvalue (as the spin operator also has the eigenvalue of 'm h_bar'). We call the 'spin' 'm' value 'm_s' and report it as the spin quantum number (one of the 4 quantum numbers). We call the 'm_L' value the magnetic quantum number as it tells us information about the angular momentum's 3D orientation (and angular momentum relates to magnetism as shown in the experiments page).
|
Looking at the picture above, one might start to notice a problem. If we keep applying the raising or lowering operator to the wave function, we are just rotating the angle momentum vector in a circle. This means that if I keep applying the same raising or lowering operator, I will eventually return back to my original m-value. There will reach a point where I apply the raising operator (rotate the angular momentum), but actually decrease the z-projection.
This is a problem. The only way to fix this problem is if the ladder operator eventually kills the wave function right at this maximum value, so that we never go over the max L_z. To do this we apply our boundary condition:
This is a problem. The only way to fix this problem is if the ladder operator eventually kills the wave function right at this maximum value, so that we never go over the max L_z. To do this we apply our boundary condition:
We will call this maximum 'm' value 'L': the angular momentum quantum number. While not directly related to the angular momentum, knowing this 'L' value informs us, by extension, about the angular momentum (we know its maximum projection on the z-axis).
We can actually use this boundary condition to find the eigenvalue of the L^2 operator. First, let us define the L^2 operator in terms of the ladder operators:
We can actually use this boundary condition to find the eigenvalue of the L^2 operator. First, let us define the L^2 operator in terms of the ladder operators:
In order to make sure everyone is following, let us review some key steps below:
12: The geometric definition of the L^2 operator
12 to 13: We replace Lx^2 + Ly^2 with a function that looks like the ladder operators (add back the difference)
13 to 14: Plug in the ladder operators and notice that the middle expression is just a commutator
14 to 15: We have previously solved for this commutator. We plug in its value
The same logic is used in equations 16 - 19 but we switch the ladder operator order. We could have also just used the commutator between then that we found in equation 4.
With this new L^2 format (in terms of the ladder operator), we can solve for its eigenvalue. To do this, we will remember that the ladder operators only rotated the angular momentum, but DID NOT change it. Therefore, it will make no difference to if we evaluated the angular momentum for the psi_top wave function (the ladder operator boundary condition). We have previously shown that the ladder operators do not change the total angular momentum (just rotates it). With this in mind, lets evaluate the total angular momentum for this boundary condition wave function:
12: The geometric definition of the L^2 operator
12 to 13: We replace Lx^2 + Ly^2 with a function that looks like the ladder operators (add back the difference)
13 to 14: Plug in the ladder operators and notice that the middle expression is just a commutator
14 to 15: We have previously solved for this commutator. We plug in its value
The same logic is used in equations 16 - 19 but we switch the ladder operator order. We could have also just used the commutator between then that we found in equation 4.
With this new L^2 format (in terms of the ladder operator), we can solve for its eigenvalue. To do this, we will remember that the ladder operators only rotated the angular momentum, but DID NOT change it. Therefore, it will make no difference to if we evaluated the angular momentum for the psi_top wave function (the ladder operator boundary condition). We have previously shown that the ladder operators do not change the total angular momentum (just rotates it). With this in mind, lets evaluate the total angular momentum for this boundary condition wave function:
In order to make sure everyone is following, let us review some key steps below:
20: We plug in our ladder operator version of the L^2 operator
20 to 21: The raising operator on the top wave function annihilates it (boundary condition)
21 to 22: We plug in our eigenvalues for the L_z operator
22 to 23: Simplify the expression
We now have an eigenvalue for the L^2 operator. The last note to make is about the bound on the quantum number 'L.' We know that 'm' scaled the integers from +/- infinity, but 'L' is actually a bounded integer. We can see in equations 27 and 23 (for the top and bottom case), the angular momentum value does not change. While the 'm' value differentiated the two cases with a +/- sign, we did not for the angular momentum 'L' value. We therefore loose half of the 'L' values (either the positive or negative ones). We arbitrarily continue on with only the positive values (just a convention). We therefore bound the 'L' value to start at the integer 0. There actually is also an upper bound for 'L' but we have not yet introduced this constraint (we will see this as we evaluate the hydrogen atom).
To summarize:
20: We plug in our ladder operator version of the L^2 operator
20 to 21: The raising operator on the top wave function annihilates it (boundary condition)
21 to 22: We plug in our eigenvalues for the L_z operator
22 to 23: Simplify the expression
We now have an eigenvalue for the L^2 operator. The last note to make is about the bound on the quantum number 'L.' We know that 'm' scaled the integers from +/- infinity, but 'L' is actually a bounded integer. We can see in equations 27 and 23 (for the top and bottom case), the angular momentum value does not change. While the 'm' value differentiated the two cases with a +/- sign, we did not for the angular momentum 'L' value. We therefore loose half of the 'L' values (either the positive or negative ones). We arbitrarily continue on with only the positive values (just a convention). We therefore bound the 'L' value to start at the integer 0. There actually is also an upper bound for 'L' but we have not yet introduced this constraint (we will see this as we evaluate the hydrogen atom).
To summarize:
|
|
|