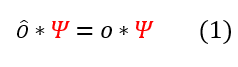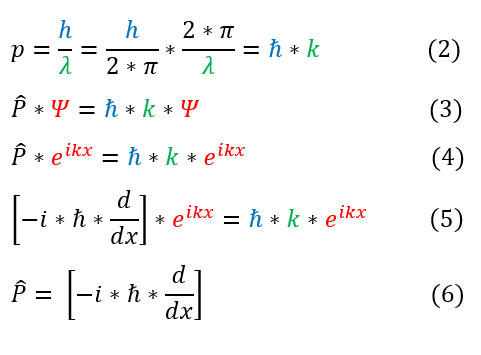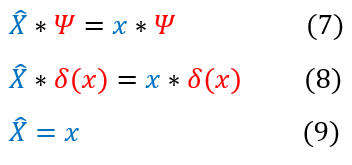Mind Network - Samuel Solomon
Introduction to Quantum Operators
The Formalism
We have shown that the wave function has no physical meaning, besides the ansatz (postulate) that the square of the wave function relates to the probability density. The wave function is only a mathematical tool and we want to start using it. One such way we can use the wave function is to act on it with operators.
Operators are defined to be functions that act on and scale wave functions by some quantum property (for example: the angular momentum operator would scale the wave function by the magnitude of the angular momentum). By scaling the wave function instead of changing it, we can easily distinguish and pull out the wave function from the expression (allowing us to determine how much the function is scaled by). This is just our mathematical choice of how we want to define operators. Because the wave function isn’t physical, the operator itself has no physical meaning as well. The only point of the operator is to mathematically show us an experimental property (usually a real one) of the atom. It extracts and relays us this information through its eigenvalue.
For clarity, let us see this written out. For some 'O' operator (generally written with a hat on top), let us pretend the atom has some 'O' property with magnitude 'o.' To find the value 'o' we would write:
Operators are defined to be functions that act on and scale wave functions by some quantum property (for example: the angular momentum operator would scale the wave function by the magnitude of the angular momentum). By scaling the wave function instead of changing it, we can easily distinguish and pull out the wave function from the expression (allowing us to determine how much the function is scaled by). This is just our mathematical choice of how we want to define operators. Because the wave function isn’t physical, the operator itself has no physical meaning as well. The only point of the operator is to mathematically show us an experimental property (usually a real one) of the atom. It extracts and relays us this information through its eigenvalue.
For clarity, let us see this written out. For some 'O' operator (generally written with a hat on top), let us pretend the atom has some 'O' property with magnitude 'o.' To find the value 'o' we would write:
Now we can easily find the value 'o' (on paper, this has nothing to do with the experimental procedure). In most cases, we usually know a particle's wave function (the function that describes the particle in space) and the operator (which we define for a given wave function). This allows us to easily extract the eigenvalue 'o' on paper.
There are a couple things to note about quantum operators:
1. An operator on psi(x) does not change the function psi(x), but rather scales it.
We cannot change the wave function because observing (let’s say) the particle’s position does not actually
change the particle. All we are doing is OBSERVING it. Scaling the wave function is just temporarily removing
the normalization (unless the constant has a magnitude of 1, in which case we call it a unitary operator)
2. Psi(x) is an eigenfunction of the operator O, yielding an eigenvalue o
The physical value we get from the operator is called the eigenvalue. The operator itself is defined for a specific basis (psi) to yield this exact physical value.
Let us work through a couple common examples:
1. MOMENTUM:
There are a couple things to note about quantum operators:
1. An operator on psi(x) does not change the function psi(x), but rather scales it.
We cannot change the wave function because observing (let’s say) the particle’s position does not actually
change the particle. All we are doing is OBSERVING it. Scaling the wave function is just temporarily removing
the normalization (unless the constant has a magnitude of 1, in which case we call it a unitary operator)
2. Psi(x) is an eigenfunction of the operator O, yielding an eigenvalue o
The physical value we get from the operator is called the eigenvalue. The operator itself is defined for a specific basis (psi) to yield this exact physical value.
Let us work through a couple common examples:
1. MOMENTUM:
In order to make sure everyone is following, let us review some key steps below:
2: The de Broglie relation for momentum rewritten in terms of h_bar and k (our desired eigenvalue)
3: Equation 1 where the O operator is the momentum operator.
3 to 4: replacing psi with the wave function we defined earlier (e^ikx)
4 to 5: A trial function is placed in the momentum operator position. When multiplied out, it checks to h_bar * k
Note that operators have no physical meaning besides for their eigenvalues produced. Hence, we cannot mathematically derive this operator relation. Our constraints for the operator function are only that the operator must be defined for a general wave function (hence, we could not add k to the operator as k is different for every wave; hence, we need to get k out of our specific wave function).
Defining an operator for a wave function works because in real applications the wave functions are generally known or approximated for a system (through a process we will later discuss). Finding a wave function is very non-trivial (and really most / basically all are approximated), but it is a good check to see for a trial wave function that this pre-defined operator does yield a correct observable.
2. POSITION:
2: The de Broglie relation for momentum rewritten in terms of h_bar and k (our desired eigenvalue)
3: Equation 1 where the O operator is the momentum operator.
3 to 4: replacing psi with the wave function we defined earlier (e^ikx)
4 to 5: A trial function is placed in the momentum operator position. When multiplied out, it checks to h_bar * k
Note that operators have no physical meaning besides for their eigenvalues produced. Hence, we cannot mathematically derive this operator relation. Our constraints for the operator function are only that the operator must be defined for a general wave function (hence, we could not add k to the operator as k is different for every wave; hence, we need to get k out of our specific wave function).
Defining an operator for a wave function works because in real applications the wave functions are generally known or approximated for a system (through a process we will later discuss). Finding a wave function is very non-trivial (and really most / basically all are approximated), but it is a good check to see for a trial wave function that this pre-defined operator does yield a correct observable.
2. POSITION:
The position in a position-space wave function has an easy eigenvalue (just the 'x' location on the particle). The one problem, for a given psi(x), 'x' can be any number from +/- infinity. This would not uniformly scale any function, except for the Dirac delta function (which is zero in every location except for at 'x'). This operator may seem a little tedious, but it is useful to solve problem so it is worth noting.
Remember that psi(x,t) is a function of x and t, which means these need to be given in order to solve the function in the first place. While this won't be discussed here, position-space wave functions can be Fourier transformed into its momentum-space counterpart. This would provide a different wave function and a different quantum operator (not the operators shown above). For those interested in other spaces (such as momentum space), a useful mathematical tool to know is if you Fourier transform a normalized wave function it preserves its norm based on Parseval's theorem.
Remember that psi(x,t) is a function of x and t, which means these need to be given in order to solve the function in the first place. While this won't be discussed here, position-space wave functions can be Fourier transformed into its momentum-space counterpart. This would provide a different wave function and a different quantum operator (not the operators shown above). For those interested in other spaces (such as momentum space), a useful mathematical tool to know is if you Fourier transform a normalized wave function it preserves its norm based on Parseval's theorem.
|
|
|