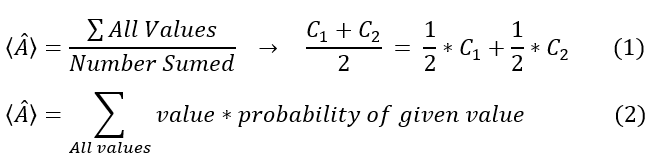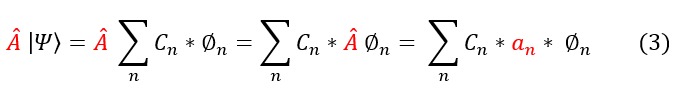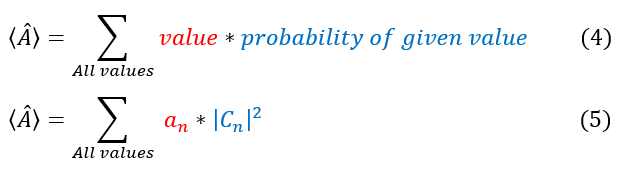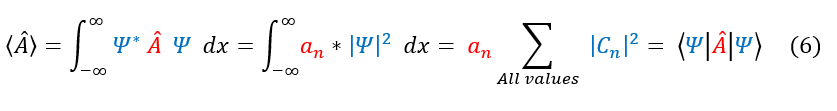When performing a quantum experiment (or any experiment for that matter), it is important to have some general sense of what the result will be. While results may vary (sometimes drastically), we can always find some average value to expect. We call this the expectation value.
To calculate the expectation / average value for quantum operators, let us revisit the general definition of average values. The average of any quantity is defined (with some example value C_1 and C_2) as:
To calculate the expectation / average value for quantum operators, let us revisit the general definition of average values. The average of any quantity is defined (with some example value C_1 and C_2) as:
Equation 2 is the general definition of the average (total sum / number summed) for an example value of C_1 and C_2. In this case, there is 1 C_1 for every C_2, and hence the probability of getting a C_1 or a C_2 is 1/2. If we had 3 C_1 for 1 C_2, then we would have 3/4 C_1 + 1/4 C_2. This weighted average approach is represented mathematically in equation 3.
Now let us translate this into quantum mechanics. Let us start with a given wave function psi, which is composed of basis functions phi. When applying an operator A to psi, the operator acts on every single basis phi, producing a series of possible eigenvalues depending on which basis we are in. This is shown visually below:
Now let us translate this into quantum mechanics. Let us start with a given wave function psi, which is composed of basis functions phi. When applying an operator A to psi, the operator acts on every single basis phi, producing a series of possible eigenvalues depending on which basis we are in. This is shown visually below:
a_n are the values we want to add up. The probability of getting an a_n value depends on the probability we are operating on its corresponding basis function phi_n. We have previously computed this probability (of being in any basis state) as |C_n|^2. Hence, the average value of an operator is given by:
We were able to previously find |C_n|^2 through normalizing the probability density function |psi|^2. Therefore, we can insert the integral of psi^2 in place of the C_n^2. This yields the average value equation below:
Just like every average value, it is important to note that the average value is not necessarily a real value. For example, the average value of 3 and 5 is 4, but 4 is not one of the initial value options. The same holds for quantum mechanics. An average value of 0 does not necessarily mean that the system can ever have a value of 0.
|
|
|
