Mind Network - Samuel Solomon
Motivation for Quantum Mechanics
Photoelectric effect
Quantum Mechanics was not an intuitive leap that physicists made one day. Rather, it was a cumulative ansatz (guess) to a series of unexplained experiments in an era of increasing experimental accuracy. One such experiment was the photoelectric effect. The photoelectric effect is simple. When high energy light is shined on a metal, electrons are ejected off (recorded as a current through a wire). In our classical picture, light is just a continuous wave.
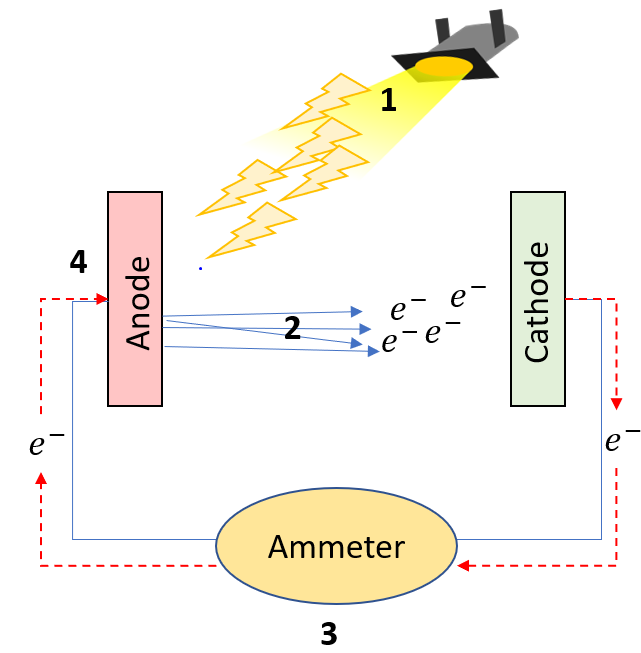
On the figure on the left:
1. High energy light is shined onto a metal surface (anode: as it will be oxidized (loose electrons) in this scenario)
2. The electrons are ejected from the anode and are quickly taken up by the cathode metal (which is reduced)
3. The ammeter measures the current. A voltmeter can also be placed to measure the potential difference.
4. The anode is then grounded (given back its electrons) through the same wire.
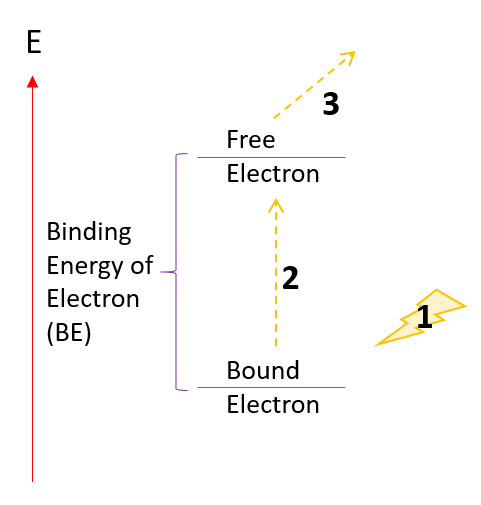
On the figure on the right:
1. Light of some energy E comes in.
2. It used some of its energy to eject the bound electron. Total energy loss is the ionization energy (IE) of electron.
3. The free electron is ejected and has the remaining energy of the photon, which is = E - IE
Altogether, this experiment makes intuitive sense. Light, like all species in this world, has energy. If it hits an electron in the right place it can transfer some of its energy to the electron and eject the electron off. The not intuitive part comes when one starts to vary some of the parameters of this experiment. Let us analyze those.
Experimental Variations:
1. Below a certain frequency of light, no electrons are ejected regardless of the intensity of light (number of particles).
a. Classical Mechanics: Light is a continuous wave. Greater intensity = more particles = more energy (WRONG)
b. Quantum Mechanics: The energy of light relates to frequency and cannot be a continuous spectrum of particles.
The classical picture has light continuously hitting the electron. However, then if you increase the number of particles, then more would hit the electron at the exact same time. Since this is not experimentally observed, the quantum picture has light in discrete bunches. Adding more particles would not mean that all light particles hit the same electron. In fact, it is very unlikely to have two light particles hitting the exact same location at once.
The classical theory of light as a wave does not hold in this experiment. Therefore, light cannot be a classical wave.
1. High energy light is shined onto a metal surface (anode: as it will be oxidized (loose electrons) in this scenario)
2. The electrons are ejected from the anode and are quickly taken up by the cathode metal (which is reduced)
3. The ammeter measures the current. A voltmeter can also be placed to measure the potential difference.
4. The anode is then grounded (given back its electrons) through the same wire.
On the figure on the right:
1. Light of some energy E comes in.
2. It used some of its energy to eject the bound electron. Total energy loss is the ionization energy (IE) of electron.
3. The free electron is ejected and has the remaining energy of the photon, which is = E - IE
Altogether, this experiment makes intuitive sense. Light, like all species in this world, has energy. If it hits an electron in the right place it can transfer some of its energy to the electron and eject the electron off. The not intuitive part comes when one starts to vary some of the parameters of this experiment. Let us analyze those.
Experimental Variations:
1. Below a certain frequency of light, no electrons are ejected regardless of the intensity of light (number of particles).
a. Classical Mechanics: Light is a continuous wave. Greater intensity = more particles = more energy (WRONG)
b. Quantum Mechanics: The energy of light relates to frequency and cannot be a continuous spectrum of particles.
The classical picture has light continuously hitting the electron. However, then if you increase the number of particles, then more would hit the electron at the exact same time. Since this is not experimentally observed, the quantum picture has light in discrete bunches. Adding more particles would not mean that all light particles hit the same electron. In fact, it is very unlikely to have two light particles hitting the exact same location at once.
The classical theory of light as a wave does not hold in this experiment. Therefore, light cannot be a classical wave.
|
|
|